Investigating Empirical ICCs

Engberg, Garde and Kreiner (EGK, 1995) investigate the measurement properties of the EG Motor Index for assessing patients mobility after a stroke. They discover that a 10 item observational instrument "can, at least to a certain extent, be regarded as unidimensional.., compatible with the concepts of regularity in recovery after stroke." Items include "Sitting balance", "Move sidewards supine" and "Sit up with legs over bed on sound side", rated 0=Totally unable, 1=With help, 2=Independent.

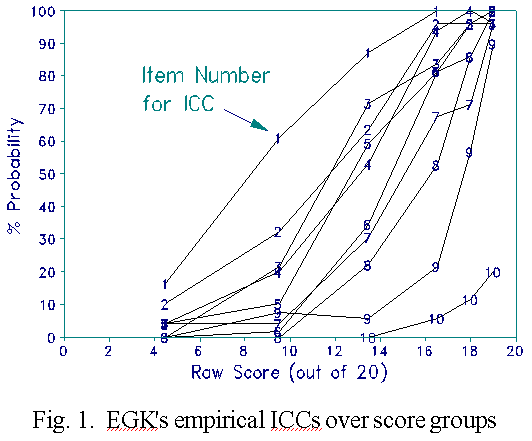
EGK do not report measures for their 180 patient assessments, nor calibrations for their 10 items. But they do report the empirical item characteristic curves [see Figure 1]. These are hard to evaluate by eye, though they do seem to have the expected ogival form.

Straight lines are easier to evaluate than curves. Figure 2 recasts the empirical values into logits which are expected to approximate linearity. The "% Probability" shown in Figure 1 is linearized by taking its logit: log_e(%/(100-%)), substituting 0.5% for 0.0% and 99.5% for 100%. The raw score in Figure 1 (which substitutes for the person measure) is linearized by taking its logit: log_e(score/(20-score)) where 20 is the maximum raw score. Figure 2 is clearer than Figure 1, but still confusing to the eye. The zig-zag form of each line provides an uninterpretable excess of detail that may merely reflect expected stochasticity in the data.

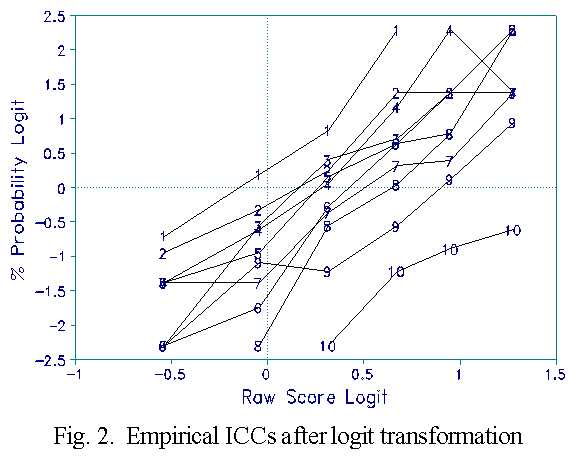
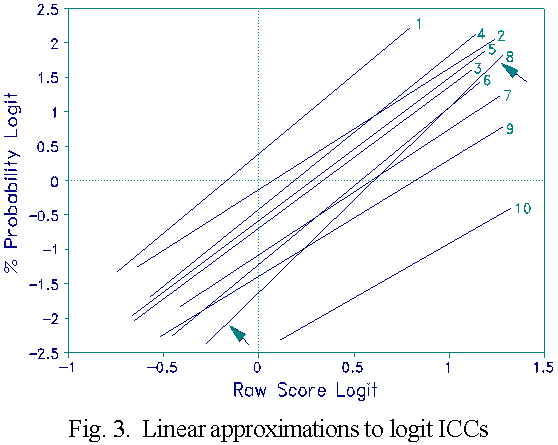
Figure 3 simplifies Figure 2 by replacing each zig-zag line with a single straight line that approximates it. If the data fit the Rasch model, the lines would be roughly parallel. In fact, the straight lines are almost parallel. Interesting deviations, such as that of item 8, can now be identified for further examination. Item 8, "Walking" is a much more athletic activity than the others listed, and so it more strongly differentiates different levels of mobility. Its steeper line signals higher discrimination. If EGK had reported local fit statistics, Item 8 would exhibit a muted INFIT mean- square, i.e., less than 1.0. This Figure also provides item calibrations at the ICC intercepts with the X-axis.

As William Fisher remarked (RMT 9:2 p. 426), perhaps their successful application of Rasch to this data will encourage EGK to use the results of their Rasch analysis to further aid in the construction of a yet more effective measuring instrument.

Engberg A, Garde B, Kreiner S (1995) Rasch analysis in the development of a rating scale for assessment of mobility after stroke. Acta Neurologica Scandinavica 91: 118-127.

Mobility after stroke: investigating empirical ICCs. Engberg A, Garde B, Kreiner S. … Rasch Measurement Transactions, 1995, 9:3 p.449

Rasch-Related Resources: Rasch Measurement YouTube Channel
Rasch Measurement Transactions & Rasch Measurement research papers - free	An Introduction to the Rasch Model with Examples in R (eRm, etc.), Debelak, Strobl, Zeigenfuse	Rasch Measurement Theory Analysis in R, Wind, Hua	Applying the Rasch Model in Social Sciences Using R, Lamprianou	El modelo métrico de Rasch: Fundamentación, implementación e interpretación de la medida en ciencias sociales (Spanish Edition), Manuel González-Montesinos M.
Rasch Models: Foundations, Recent Developments, and Applications, Fischer & Molenaar	Probabilistic Models for Some Intelligence and Attainment Tests, Georg Rasch	Rasch Models for Measurement, David Andrich	Constructing Measures, Mark Wilson	Best Test Design - free, Wright & Stone Rating Scale Analysis - free, Wright & Masters
Virtual Standard Setting: Setting Cut Scores, Charalambos Kollias	Diseño de Mejores Pruebas - free, Spanish Best Test Design	A Course in Rasch Measurement Theory, Andrich, Marais	Rasch Models in Health, Christensen, Kreiner, Mesba	Multivariate and Mixture Distribution Rasch Models, von Davier, Carstensen
Rasch Books and Publications: Winsteps and Facets
Applying the Rasch Model (Winsteps, Facets) 4th Ed., Bond, Yan, Heene	Advances in Rasch Analyses in the Human Sciences (Winsteps, Facets) 1st Ed., Boone, Staver	Advances in Applications of Rasch Measurement in Science Education, X. Liu & W. J. Boone	Rasch Analysis in the Human Sciences (Winsteps) Boone, Staver, Yale	Appliquer le modèle de Rasch: Défis et pistes de solution (Winsteps) E. Dionne, S. Béland
Introduction to Many-Facet Rasch Measurement (Facets), Thomas Eckes	Rasch Models for Solving Measurement Problems (Facets), George Engelhard, Jr. & Jue Wang	Statistical Analyses for Language Testers (Facets), Rita Green	Invariant Measurement with Raters and Rating Scales: Rasch Models for Rater-Mediated Assessments (Facets), George Engelhard, Jr. & Stefanie Wind	Aplicação do Modelo de Rasch (Português), de Bond, Trevor G., Fox, Christine M
Exploring Rating Scale Functioning for Survey Research (R, Facets), Stefanie Wind	Rasch Measurement: Applications, Khine	Winsteps Tutorials - free Facets Tutorials - free	Many-Facet Rasch Measurement (Facets) - free, J.M. Linacre	Fairness, Justice and Language Assessment (Winsteps, Facets), McNamara, Knoch, Fan

Forum

Rasch Measurement Forum to discuss any Rasch-related topic

Go to Top of Page
Go to index of all Rasch Measurement Transactions
AERA members: Join the Rasch Measurement SIG and receive the printed version of RMT
Some back issues of RMT are available as bound volumes
Subscribe to Journal of Applied Measurement

Go to Institute for Objective Measurement Home Page. The Rasch Measurement SIG (AERA) thanks the Institute for Objective Measurement for inviting the publication of Rasch Measurement Transactions on the Institute's website, www.rasch.org.

The URL of this page is www.rasch.org/rmt/rmt93h.htm

Website: www.rasch.org/rmt/contents.htm